How symmetries, or lack thereof, shape the universe around

September 27, 2024 by xavipondi

However, sometimes symmetries can be (spontaneously) broken, leading to even more interesting effects. A classic example of this is a pencil. A pencil standing on its tip has rotational symmetry; all directions are the same. However, its "minimum energy" state is not on its tip but on the ground (see Figure 1). Once the pencil falls into one of the possible ground positions, the symmetry is broken, as the pencil has chosen a particular position.

Figure 1. A pencil on its tip will eventually fall into the ground, with all the positions being equivalent. Once it falls into a particular position, the symmetry is broken by this choice. Source: Nobel Prize website.

Particle physics exhibits a similar type of process as the pencil, although a bit more complicated, which is known as the Higgs mechanism. In gravity, objects far from a massive object have potential energy, close to the earth's surface the higher you go the more potential energy you have. Similarly, the Higgs field has a potential, but in this case the relation is not as simple. The dependence of the field's energy with respect to the values it can take resembles a Mexican hat, see Figure 2. In the case of gravity, the minimum is at the surface, where the object cannot fall, but in the case of the Higgs, the minimum energy state is not where the field is zero, but at a certain value.

We can see, as for the example of the pencil, that all the minima are at an equal distance from the center, however, the minimum energy state of the Higgs will be only one (as seen in Figure 2). As for the pencil, the Higgs picks a point and the symmetry is said to be spontaneously broken.

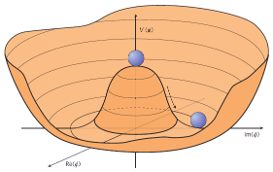
Figure 2. The Higgs potential has the shape of a Mexican hat. The position on the tip of the hat is unstable, as it has more potential energy and falls into a less energy state, choosing one of the equivalent ground states. Source: The standard theory of particle physics: essays to celebrate CERN's 60th anniversary.

This has an interesting consequence for the Standard Model of particle physics: it gives mass to some particles. We classify particles into fermions and bosons. Fermions are what we call "matter" as they build atoms, while bosons mediate the forces, for instance, the photon is the mediator of electromagnetism. A summary of all the building blocks of the Standard Model can be found in Figure 3.

The Higgs is coupled to the fermions of the Standard Model, so when the field chooses one of the minimums, the fermions propagate on a "background" that is not zero. Imagine it as if you were moving in a medium with a certain density, this density slows you down, making you feel that there is a force dragging you. Fermions move now in such a kind of medium, which is given by the Higgs field value at the minimum.

So, in this way, the Higgs mechanism explains the fermion masses of the Standard Model particles. Something that remains unanswered, and is one of the current research directions in theoretical particle physics, is why the Higgs interacts so differently with the fermion families of the Standard Model. As can be seen in Figure 3, the SM has three columns of fermions, each generation or family, is a heavier copy of the previous. And, although the Higgs mechanism explains why they have a mass, it does not explain why the mass difference between the top-quark is 70.000 times heavier than the u-quark.

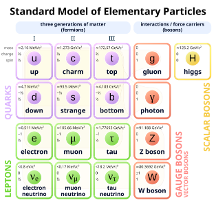
Figure 3. Known fundamental particles of the Standard Model. Source: wikipedia.

The Higgs boson also gives mass to the mediators of the weak force, known as the W- and Z- bosons. These mediators also couple to the Higgs, while the mediators of electromagnetic and strong interactions, the photon and the gluon, don't and remain massless. The consequence of massive mediators is that the weak force has a short range of interaction, while electromagnetism has an infinite range and can act at arbitrary distances.

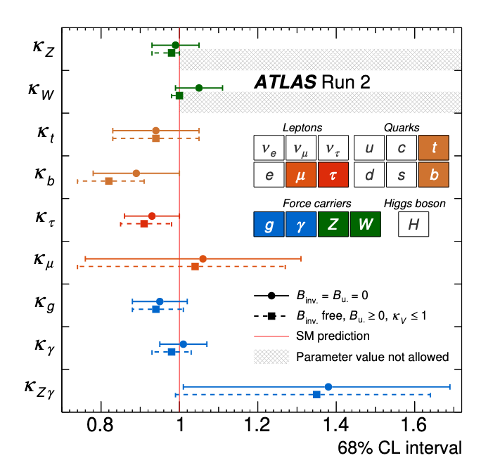
Figure 4. Measurements of the Higgs properties. The parameters Κ parametrise how close the experimental measurements of the Higgs boson are to the theory predictions. A value of 1 means it is compatible with the theory. Source: PDG.

The last piece, which was found in 2012 at the LHC was the Higgs boson. This particle is the excitation of the field from the minimum, that is what happens if the field is not at its low energy value. This particle interacts in a very specific way with the particles of the Standard Model that we can predict. So, currently, ATLAS and CMS experiments are studying all the properties of the Higgs to understand if it behaves as we expect it or if there is something we still do not understand of this mechanism. We see in Figure 4 the experimental values of the "Κ" parameters, which tell us how similar to the Standard Model the measurements are. A measurement of 1 means that it is compatible with how we expect the Higgs boson to behave.

As we can see in Figure 4, the Higgs boson closely resembles what we expect from the Standard Model. However, there are still some unanswered questions. Something that has bothered theoretical physicists for a long time is the mass of the Higgs. This parameter of the theory is not stable, so if there are new heavy particles above the Standard Model, the Higgs mass would tend to be close to this heavy mass scale. This is produced via what we call "radiative corrections", which are virtual particles that are created and then recombined into the Higgs giving a contribution to its mass, see Figure 5. This is known as the Higgs hierarchy problem.

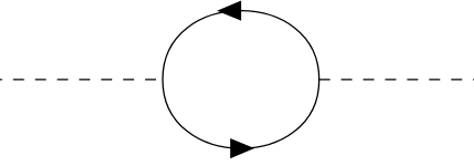
Figure 5. Diagram in which the Higgs emits two virtual particles which are then recombined contributing to the Higgs mass. 

Solutions to this problem proposed other particles that would compensate for these radiative corrections, such as supersymmetry (SUSY). In this theory, we have as many fermions and bosons, and in exact SUSY the contributions from a fermion and a boson with the same mass cancel. In this way, the mass of the Higgs would be protected. The only problem is that we have not seen the supersymmetric partners of the particles of the Standard Model, so the question remains unanswered.

The Higgs particle discovery is a story of the success of a theory proposed 50 years ago which was recently proven correct. However, still, some unanswered questions remain open, so perhaps this last piece of the Standard Model may be the first one to a new paradigm of particle physics.

- Xavier Ponce